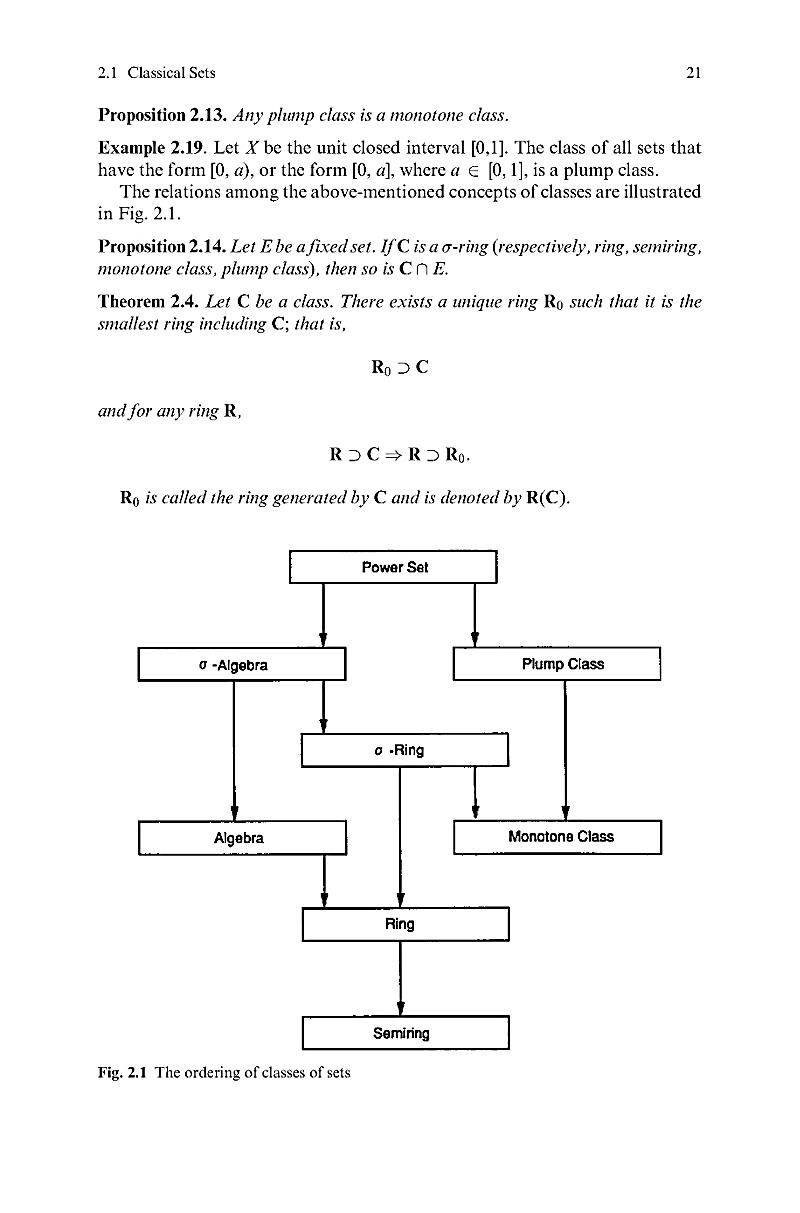
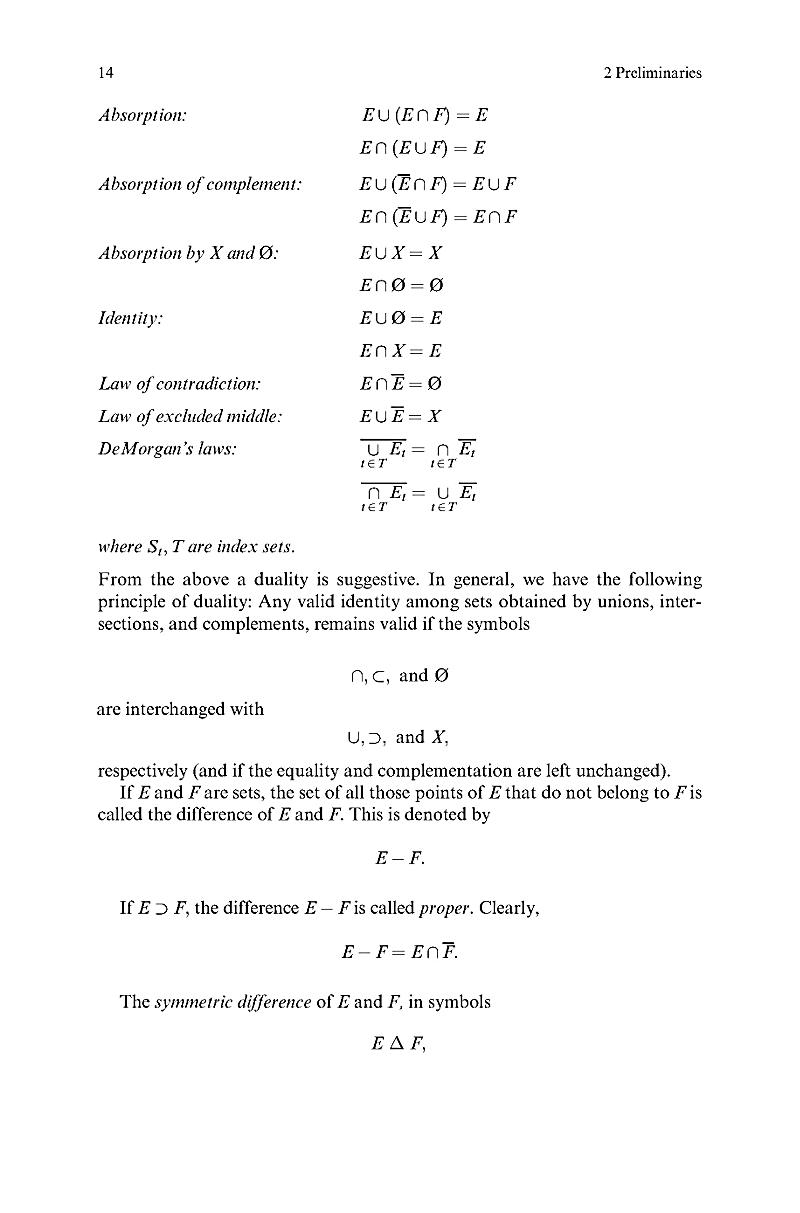
Definition of Lebesgue Measurable for Sets with Finite Outer Measure Remove Restriction of Finite Outer Measure (Rn, L, Lambda) is a Measure Space, i. , L is a Sigmaalgebra, and Lambda is a Measure Measure Theory. From Wikibooks, open books for an open world. Jump to navigation Jump to search. This book requires that you first read. Lectures on Measure Theory and Probability by H. Pitt Notes by Raghavan Narasimhan No part of this book may be reproduced in any form by print, microlm or any other means with Measure theory is a bit like grammar, many people communicate clearly without worrying about all the details, but the details do exist and for good reasons. In mathematics, more precisely in measure theory, an atom is a measurable set which has positive measure and contains no set of smaller but positive measure. A measure which has no atoms is called nonatomic or atomless. Topics covered include a quick review of abstract measure theory, theorems and differentiation in Mn, lower Hausdorff measures, area and coarea formulas for Lipschitz mappings and related changeofvariable formulas, and Sobolev functions and functions of bounded variation. Measure algebras are a coherent way to ignore the sets of measure 0 in a measure space [P, Sect. Many of the difficulties of measure theory and all the pathology of the subject arise from the existence of sets of measure zero. The algebraic treatment gets rid of this source of. An Introduction to Measure Theory. This is a preliminary version of the book An Introduction to Measure Theory published by the American Mathematical Society (AMS). This preliminary version is made available with But measure theory is simply concerned with measures, which are (intuitively speaking) a kind of description of size, and there's no reason that you wouldn't care about how big things are in number theory. In fact, number theory is the study of the structure of the integers, and a significant part of structure is how big things are. Measure theory studies ways of generalizing the notions of lengthareavolume. Even in 2 dimensions, it might not be clear how to measure the area of the following fairly tame shape: much less the area of even weirder shapes in higher dimensions or different spaces entirely. Measure Theory 1 Measurable Spaces A measurable space is a set S, together with a nonempty collection, S, of subsets of S, satisfying the following two conditions. Geometric measure theory has become increasingly essential to geometry as well as numerous and varied physical applications. The third edition of this leading textreference introduces the theory, the framework for the study of crystal growth, clusters of soap bubbles, and similar structures involving minimization of energy. 2010 MEASURE THEORY ALP Introduction In mathematics, more specically in measure theory, a measure on a set is a systematic way to assign to each suitable subset a number, as the size of the subset. Content: The modern notion of measure, developed in the late 19th century, is an extension of the notions of length, area or volume. Objectives: To gain understanding of the abstract measure theory and definition and main properties of the integral. A measure space is denoted by X; M; ), where X is the space of points, M is the algebra of measurable sets, and is the measure, dened on M. A measure on a topological space for which the measurable sets is the Borel The term Lebesgue integration can mean either the general theory of integration of a function with respect to a general measure, as introduced by Lebesgue, or the specific case of integration of a function defined on a subdomain of the real line with respect to Lebesgue measure The measuretheoretic strategy then results in attentiongrabbing purposes and quite a number issues that come with the development of the Lebesgue degree on Rn (metric area approach), the BorelCantelli lemmas, directly degree thought (the Lebesgue integral). during this concise textual content, a few functions to chance are packed into the. Measure theory is the study of measures. It generalizes the intuitive notions of length, area, and volume. Technically, a measure is a function that assigns a nonnegative. Measure theory is a bit like grammar, many people communicate clearly without worrying about all the details, but the details do exist and for good reasons. Measure theory and probability Alexander Grigoryan University of Bielefeld Lecture Notes, October 2007 February 2008 Contents 1 Construction of measures 3 Measure theory is the study of measures. It generalizes the intuitive notions of length, area, and volume. The earliest and most important examples are Jordan measure and Lebesgue measure, but other examples are Borel measure, probability measure, complex measure, and Haar measure. Concrete examples of transformations which preserve a finite measure and ones which preserve an infinite measure exist in the literature. It's known that a basic difference exists between. Economics 245A Introduction to Measure Theory The goal of this lecture is to take the axioms of probability, which are introduced as the basis for statistical theory, and relate them to measure theory. Atom (measure theory) In mathematics, more precisely in measure theory, an atom is a measurable set which has positive measure and contains no set of smaller positive measure. A measure which has no atoms is called nonatomic or atomless. Geometric Measure Theory: A Beginner's Guide, Fifth Edition provides the framework readers need to understand the structure of a crystal, a soap bubble cluster, or a universe. The book is essential to any student who wants to learn geometric measure theory, and will appeal to researchers and mathematicians working in the field. Mostly aggregating many classical branches of measure theory the aim of the Handbook is also to cover new fields, approaches and applications which support the idea of measure in a wider sense, e. the ninth part of the Handbook. In this video, I discuss the intuition behind measures, and the definition of a general measure. I also introduce the Lebesgue Measure, without proving that it is indeed a measure. P(X), the collection of all subsets of X, is a algebra of subsets of X. The fA XjAis countable or Acis countablegis a algebra of subsets of. Measure Theory Ariel Yadin Lecture 1: Introduction 1. Measuring things Already the ancient Greeks developed a theory of how to measure length, area, and volume and area of 1; 2 and 3 dimensional objects. in Rdfor d 3) it stands to reason that the \size or \measure of an object must satisfy some basic Measure theory and integration are presented to undergraduates from the perspective of probability theory. The first chapter shows why measure theory is needed for the formulation of problems in probability, and explains why one would have been forced to invent Lebesgue theory (had it not already existed) to contend with the paradoxes of large numbers. A connection between a classical puzzle about rational numbers and what makes music harmonious. Measure theory is a classical area of mathematics born more than two thousand years ago. Nowadays it continues intensive development and has fruitful connections with most other fields of mathematics as well as important applications in physics. Measure theory is a classical area of mathematics that continues intensive development and has fruitful connections with most other fields of mathematics as well as important applications in physics. 2 Lecture: Invariant Measures 1. Let U Rn be an open set and let F (t; x) a dierentiable function of I U, where I R is an open neighbourhood of 0. Let us try to compute the derivative d dt ts Z t (U) F (t; x)dx: Applying the change of variables formula, we have In analysis: Measure theory A rigorous basis for the new discipline of analysis was achieved in the 19th century, in particular by the German mathematician Karl Weierstrass. Modern analysis, however, differs from that of Weierstrasss time in many ways, and the most obvious is the level of formalized by Kolmogorov (1933), measure theory provides the foundation of probability. Measures are important not only because of their intrinsic geometrical and probabilistic signicance, but because they allow us to dene integrals. To such a person I would admit that (currently) the applications of measure theory in statistics seems largely relegated to researchers studying new types of data and doing methodological research; however, there is a growing number of tools that actually use measure theory concepts directly in the analysis of realworld data sets. The Geometric Measure Theory and its Connections Conference at the University of Helsinki, 4 8 June 2018, focuses on recent developments and breakthroughs in geometric measure theory, with emphasis on connections to adjacent fields, in particular harmonic analysis and dynamical systems. develop a general measure theory which serves as the basis of contemporary analysis and probability. In this introductory chapter we set forth some basic concepts of measure theory, which will open for abstract Lebesgue integration. Algebras and Measures Throughout this course Semialgebras [. Roughly speaking, a semialgebra over a set is a class that is closed under intersection and semi closed under set difference. Since these restrictions are strong, it's very common that the sets in it have a defined characterization and then it's easier to construct measures over those sets. Measure Theory About the Course. This is a course on the concepts of Measure and Integration. Normally, this is a core course for M, . Mathematics and Statistics students. The concepts find applications in advance Analysis Courses, Signal Processing, Financial Mathematics courses. Brief description: In Measure Theory we look carefully at various ways to measure the size of a set. The theory makes rigorous the notions of length, area and volume, and generalises these notions. The theory makes rigorous the notions of length, area and volume, and generalises these notions. Real Analysis is the third volume in the Princeton Lectures in Analysis, a series of four textbooks that aim to present, in an integrated manner, the core areas of analysis. Here the focus is on the development of measure and integration theory, differentiation and integration, Hilbert spaces, and Hausdorff measure and fractals. Measure Theory: lt; p In mathematical analysis, a measure on a set is a systematic way to assign a number World Heritage Encyclopedia, the aggregation of the largest online encyclopedias available, and the most definitive collection ever assembled. Questions related to measures, sigmaalgebras, measure spaces, Lebesgue integration and the like. Probability and Measure Theory, Second Edition, is a text for a graduatelevel course in probability that includes essential background topics in analysis. It provides extensive coverage of conditional probability and expectation, strong laws of large numbers, martingale theory, the central limit theorem, ergodic theory, and Brownian motion. Economics 204 Lecture Notes on Measure and Probability Theory This is a slightly updated version of the Lecture Notes used in 204 in the summer of 2002. PREFACE My main purpose in this book is to present a unified treatment of that part of measure theory which in recent years has shown itself to be most useful for its applications in modern analysis..